3
$\begingroup$

Question from new ham here, regarding ways of matching impedances between a feedline and an antenna.

Is it possible to fashion a broadband HF impedance matcher from a length of windowed twinlead, in which the size of the cutout windows along the length of the matching section is smoothly varied from one end to the other?

Alternatively, is this possible by using a length of twinlead in which the distance between the conductors is smoothly varied from one end of the line to the other?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Although not ideas as you suggest, the G5RV antenna uses a windowed transmission line (300, 450, 600) as a means of helping a non-resident antenna match. You should google G5RV antennas and take a look at the design principles. $\endgroup$
    – K7PEH
    Commented May 31, 2020 at 4:47
  • 1
    $\begingroup$ I have and I do understand them, including the idea that the ladder line feeder becomes part of the antenna; in my garage I have the components of a G5RV ready to construct, I just wanted to know if impedance matching can be improved by eliminating discontinuous changes in impedance along a transmission line. $\endgroup$
    – niels nielsen
    Commented May 31, 2020 at 4:51

1 Answer 1

Reset to default
3
$\begingroup$

According to Vincent D. Matarrese's Master's thesis, "Tapered radio frequency transmission lines" (Portland State University, 1992), there is a long history of using tapered-impedance transmission lines in matching systems. It's much too big a topic to review in an SE post, but the thesis is loaded with references for exploration.

The Delta match is an example of the application of this technique:

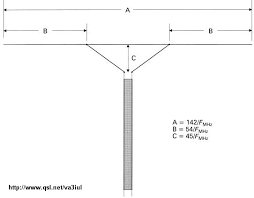
enter image description here

It has been described in the ARRL Antenna Book for decades. PA3HBB gives equations that can be used to construct a delta match for a given matching situation.

We can begin to consider the prospects for your proposal by evaluating the impedance of bare-wire twinlead and fully-immersed twinlead. The impedance of a two-parallel-wire transmission line is:

$$Z_0=\frac{276\Omega}{\sqrt{\epsilon_r}}log_{10} \frac{D}{d}$$

valid for $(\frac{D}{d}>10)$, where $\epsilon_r$ is the relative permittivity of the dielectric material between the wires, $d$ is the wire diameter and $D$ is the spacing between them. For window line, the wires are not completely immersed in the dielectric material, so the lowering of the line's impedance is considerably reduced.

Using Polyethylene's $\epsilon_r$ of 2.5 as an example, the impedance of 18-ga conductors (d=0.0403-in) spaced 1-in apart would vary between $385\Omega$ for bare wires and $243\Omega$ if the wires were fully immersed in the dielectric. Even without "windows" in the dielectric, its effect is probably ameliorated by at least a factor of two, reducing the impedance variation to about $344\Omega$, which wouldn't provide very much impedance transforming capability.

As theorized by the OP, both the spacing and the quantity of dielectric material between the wires could be varied to increase the impedance range. Materials with higher $\epsilon_r$ would increase the impedance variation, but their cost-effective application to this problem seems challenging.

Improve this answer
$\endgroup$
2
  • $\begingroup$ upvoted and accepted. Thanks for your most useful answer! -Niels $\endgroup$
    – niels nielsen
    Commented May 31, 2020 at 18:05
  • 1
    $\begingroup$ Thanks for asking a good question, Niels. Too often, questions like these go unasked, so its a pleasure to dredge through the archives and run through some numbers. Discussions like these can also lead to new ideas, so keep 'em coming! $\endgroup$
    – Brian K1LI
    Commented May 31, 2020 at 20:28

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .