No, it's not possible. The electric field vector can only point in one direction at a time, so there's no way it could simultaneously rotate in two directions.
However it is possible to consider any possible polarization as a superposition of left- and right-handed circular polarization.
When there are multiple radiation sources, either because there are multiple transmitters or because the same signal is being received through multiple paths, then the result at the receiver is the addition of each source. Adding two circular polarizations of opposite chirality together produces a linear polarization.
This can be shown graphically as a 3D parametric plot:
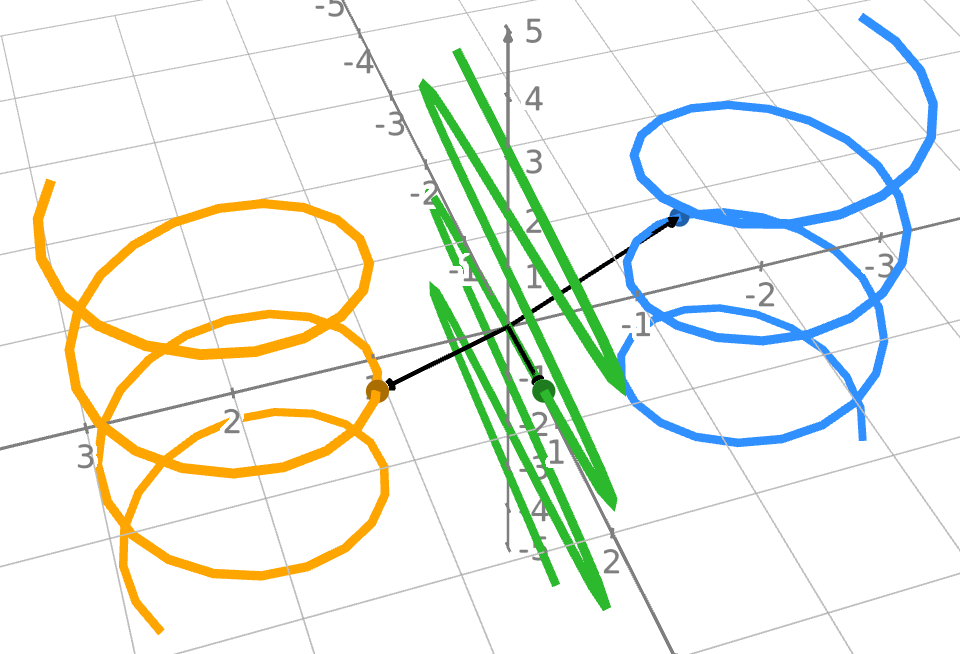
(editable source)
On the left in yellow, we have:
$$
\left\{
\begin{aligned}
x(t) &= \cos(t) \\
y(t) &= \sin(t)
\end{aligned}
\right.
$$
On the right in blue we have:
$$
\left\{
\begin{aligned}
x(t) &= -\cos(t) \\
y(t) &= \sin(t)
\end{aligned}
\right.
$$
And the middle in green is the addition of these two:
$$
\left\{
\begin{aligned}
x(t) &= \cos(t) - \cos(t) \\
y(t) &= \sin(t) + \sin(t)
\end{aligned}
\right.
$$
It's pretty plain to see this simplifies to $x(t) = 0$ as the opposite electric fields along the x axis cancel each other.
As the two sources change in relative phase, the plane of the resulting linear polarization rotates:
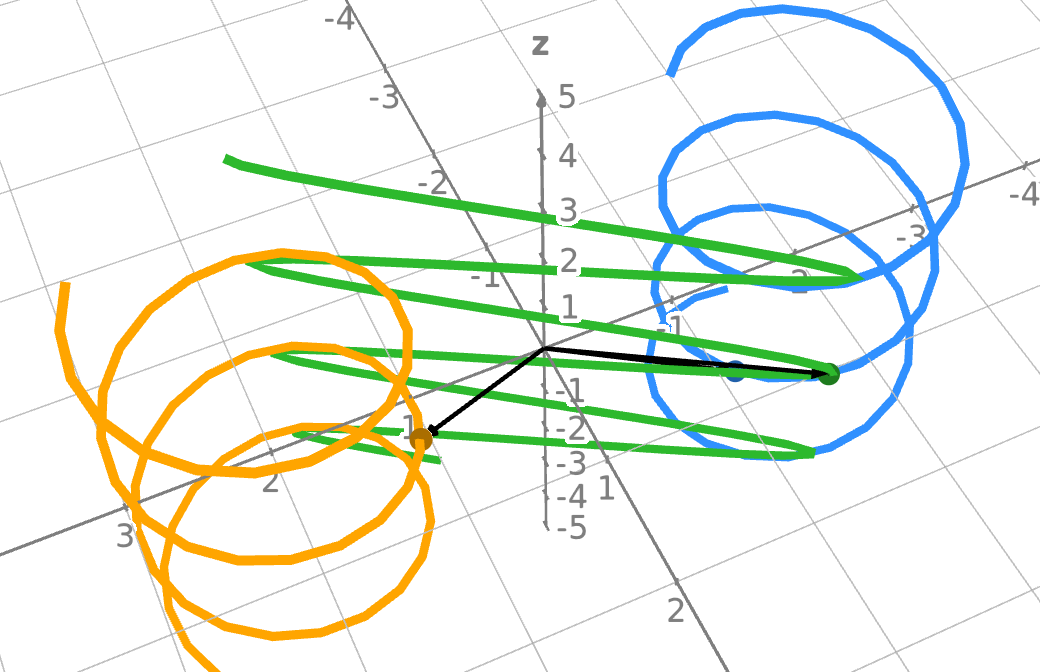
(editable source)
Here, green is showing
$$
\left\{
\begin{aligned}
x(t) &= \cos(t+2) - \cos(t) \\
y(t) &= \sin(t+2) + \sin(t)
\end{aligned}
\right.
$$
While it's not so immediately obvious to see the cancellation, it remains true that opposite helices like this will cancel each other in some plane, as long as they are equal in amplitude.
If the two sources are not equal in amplitude, then the result is elliptical polarization:
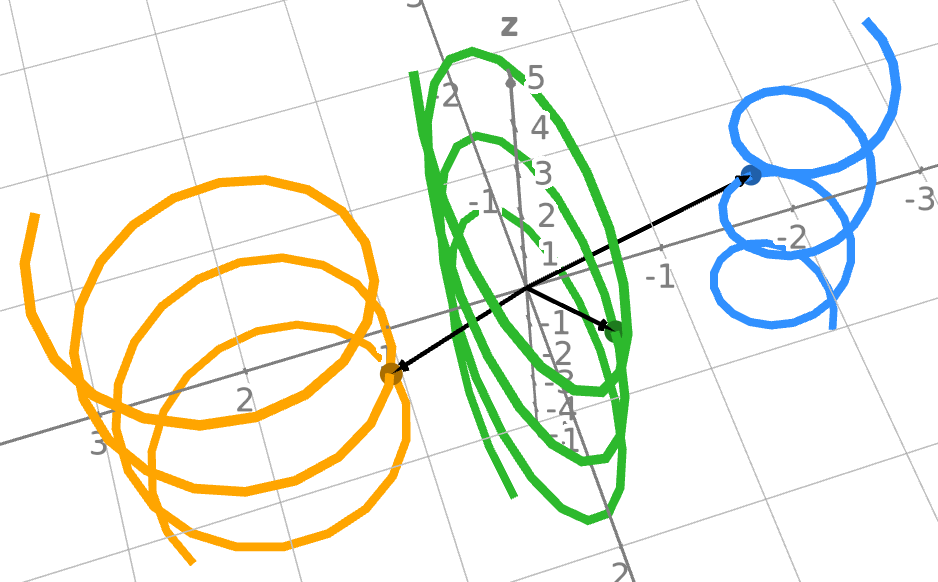
(editable source)
It is true that the ionosphere is time-variant, and so at one time the signal as received may be left-handed, and some time later right-handed. But is impossible for it to be both at the same time, although one could consider a linear polarization to be the superposition of both circular polarizations in equal amplitude.
The problem is an ionospheric channel does not guarantee equal amplitude. Thus, a circularly polarized receive antenna will still be subject to fading as the signal randomly wanders between left- and right-handed chirality, as well as linear and all the points between (elliptical polarizations).



