First of all, you need to define what your "reception percentage" would really mean to you: is it the bandwidth at which the antenna is still at least half as eefficient as at its optimum frequency? That'd be the 3dB bandwidth. There's other measures, which often are better suited for any particular thing you want to do. For example, for transmitters, often the bandwidth at which the reflection coefficients drop e.g. below -10 dB is relevant (because you might not want to reflect back more than 1/10 of the power into the transmit amplifier, for example).
Having figured out what you mean with that:
That depends on the construction of these antennas!
- in the monopole case, the only "easily adjustable" variable is the radius of the conductor. The higher the diameter, the wider the bandwidth, but these things become pretty specific to things like ground plane conductivity. I'll be honest, a "whip" is not going to be straight enough to apply any closed-form formula well enough. OK, you can apply the formula, but your result will differ from reality enough that you could as well guess. So, measure the beast! VSWR over different frequencies is the usual measurement you make.
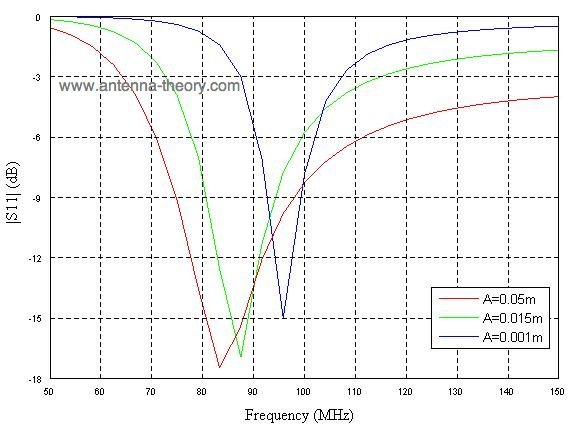
Here's a typical graph of monopole reflection over frequency, assuming differently thin conductor ($A$ is the radius):. This is all the same monopole length!
Here, the lower the better: an |S11|of -16 dB, for example, means that only 1/40 of the energy put into the antenna at that frequency gets reflected back into the source. At 0 dB, all of the energy is reflected back and the antenna doesn't act as antenna at all.
Antennas are reciprocal: As good as it is for transmit, it is for receive.
Note that you need to multiply the $A$ from the graph by roughly seven to get similar results for a monopole for 14 MHz.
Nothing really changes the fact that you won't do much above maybe 15% of center frequency as 3 dB bandwidth for a monopole antenna.
- A discone for 100 MHz will usually be a shortened discone made from radiator elements. So, yeah, another thing that will be hard to calculate, but likely quite possible to simulate (discones are typically not dependent on external ground planes as much).