Does antenna array structure effect overall radiation resistance?

Question

If we consider a basic half wave dipole array does the radiation resistance of array depend on distance between two elements and phase we feed?

Answer 1

Yes, element spacing and geometry most definitely has an effect on radiation resistance in the real world. Radiation resistance is essentially that part of the signal which is not lost in some other fashion, be it resistance of the elements, the feed line, the ground, etc. In free space, the difference either spacing or phasing make would be miniscule (or possibly zero, I'm limited in my ability to confirm that by the modeling software I use), but when you take in to account the lossy nature of an antenna's surroundings and the system attached to it, the difference can be considerable.

The precise way in which the radiation resistance will change, however, is highly situation dependent. Additional elements can effectively increase the physical aperture of the antenna, which can increase radiation efficiency (and necessarily radiation resistance). The spreading of current over a larger physical area can reduce losses in the ground, for instance.

In the case of parasitic arrays, the spacing of the elements would determine how effectively the parasitic elements interacted with the driven element, changing the amount of energy they re-radiate. The electrical length of the parasitic elements would also come in to play, as a very high impedance would result in a smaller interaction with the field around it.

In a driven array such as an LDPA, the degree to which the additional elements changed radiation resistance would depend on their own impedance relative to each other, one element with a very good impedance match and another with a poor impedance match to the transmitter would result in one element radiating a majority of the power.

An extreme example of spacing and phasing impacting radiation resistance in driven arrays can be seen in balanced feedline or "ladder line". If we imagine a theoretically perfect balanced feed line that is terminated with a perfectly matched resistive load on the far end, 100% of the power that is fed in to the feed line will be dissipated by that resistive load. The fields of the two conductors are precisely equal and opposite, perfectly canceling each other, so there can be no radiation, therefore the radiation resistance is zero.

If we move this system from our theoretically perfect example to something as close to perfect as we can get in the real world, we can see that the power would be dissipated mostly by the load, as well as some resistance in the conductors, but there would now be a third component. The two conductors are some tiny fraction of a wavelength apart, they are likely very slightly different in length, width, or resistance, and they can't inhabit exactly the same space, so the fields can not perfectly cancel in all directions. Some minute fraction of the power we feed in is now being radiated. The worse the phase match between the two conductors at an arbitrary point along their length, the more power is radiated. The radiation resistance is increasing as you change their relative phase or amplitude at a given point in the length.

When it comes to spacing, in our theoretically perfect example, we could move our conductors arbitrarily far apart and as long as the match to the load was still perfect, the radiation resistance would still be zero. If you were to move arbitrarily far away from the two conductors, their opposing fields would appear to occupy the same space, resulting in perfect cancellation.

In the real world, however, distance between the conductors does have an impact. Because the two conductors must exist in a medium which is not perfectly uniform in all directions, the two conductors will interact unequally with objects around the feed line. This means that a conductive object nearer to one conductor of the feed line not only interacts more strongly with that conductor than the other, resulting in induction of current on the object which is not opposed by any other field and thus can be radiated, it also couples more power out of the nearer conductor, resulting in an imbalance of the feedline, meaning that the feed line itself can now radiate. The radiation resistance has climbed from very nearly zero, to some appreciable fraction of the total system impedance.

The further apart you move the conductors, the more likely you are to have objects that interact strongly with only one conductor.

This same basic idea can be applied to any array, and demonstrates why the effect of phasing and spacing would be so highly dependent on real-world circumstances.

Answer 2

An antenna is effectively a transformer between the impedance of free space (377Ω) and whatever the feedpoint impedance is. For any antenna which has only negligible losses, and no reactive impedance at the feedpoint, the feedpoint impedance is equal to the radiation resistance.

Consider this model:

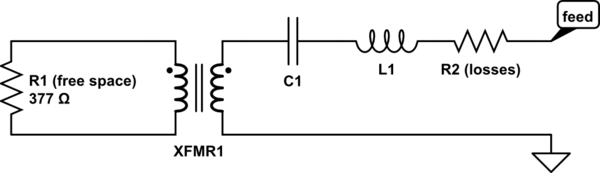
^{simulate this circuit – Schematic created using CircuitLab}

Any power put into R1 is radiated. But the impedance of that resistor is transformed by the antenna, represented by the transformer and reactive components. Additionally we have antenna losses. So when we ask what the "radiation resistance" is, really we are asking what R1 looks like from the feedpoint, through whatever impedance transformations the antenna might make.

In practice, we try to tune the reactance of the antenna (represented by C1 and L1) to 0Ω, and R2, which represents losses in the antenna, is negligibly small. So when losses are negligible and the feedpoint impedance has no reactance, then the impedance seen at the feedpoint is also the radiation resistance.

You probably already know that different antennas have different feedpoint impedances. For example, a dipole is about 72Ω, while a vertical is half that, 36Ω.

When you introduce arrays, the coupling between the arrays introduces additional reactances into the antenna. This is why Yagis (a passively driven array) usually require some additional matching network or trick (like a folded dipole driven element) to get their feedpoint impedance, and thus radiation resistance close to 50Ω. Actively driven arrays are no different.

So essentially, if your antenna requires a matching network, and it's not because you've introduced losses, it's probably because the antenna has changed the radiation resistance in an undesirable way. And if you consider the matching network as part of the antenna, then you've probably changed the radiation resistance to 50Ω.