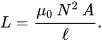I need to know one thing about making a small loop antenna using the LC and coil inductance formula.
The solenoid inductance formula is:
Where, l is the length of the coil and N is the number of turns. So, does that mean that the turns of a loop can't be overlapped?
If I need to use 20 meter long wire to wrap around a square plastic frame of 20 cm each side with a width of 2 cm, and if the wire is 1 mm thick and doesn't fit after a few turns then can I wrap the wire over the previous turns?
I need one more clarification:
A perfect resonant antenna is the one that has 1/4 of the wavelength. So, if I need to receive the 850 kHz using a loop antenna, then as per the formula, I will need a (300/0.85 = 356/4 = 88 meter) long wire.
Instead of using it as a long wire antenna, if I wrap it around a plastic square frame of any size, then will it work as a loop antenna?