Solid angle for different E/H plane beamwidths

Question

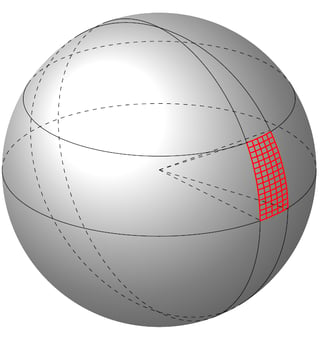
The solid angle formula calculates the surface area on a unit sphere, from projecting a rectangular patch onto the surface of a sphere.

^{Image source}

This is calculated using the azimuth angle $\phi$ and the elevation angle $\theta$:

$$\Omega = \int_{0}^{\phi} \int_{0}^{\theta} \sin{\theta'} d\theta' d\phi $$

However an antenna beam pattern will project a curved patch onto a sphere.

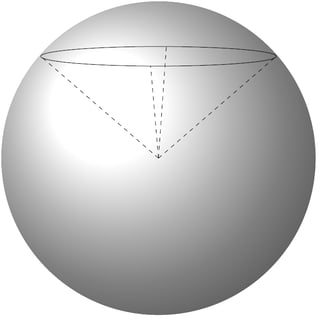
Case 1: Circle projection

First consider a linearly polarized, symmetrical parabolic dish antenna. This ideal geometry ensures the E/H planes have the same beamwidth. A circle is projected onto the unit sphere.

Note this is equivalent to the surface area of a hemisphere between the circle and the sphere.

$$\Omega = \int_{0}^{2\pi} \int_{0}^{\theta} \sin{\theta'} d\theta' d\phi = 2\pi(1-\cos{\theta})$$

Case 2: Ellipse projection

Now consider any linearly polarized antenna, such that the E/H planes have different beamwidths. I presume this would be projecting an ellipse onto the unit sphere.

I presume the solid angle can be calculated from the surface area of a semi-ellipsoid. A solid angle is a fraction of the surface area of the unit sphere, the ellipsoid is not coincident with a sphere (other than the trivial case), so this can't be correct.

Some questions:

• How can the solid angle formula be used to derive the (elliptical) solid angle of an antenna with a beamwidth of $\phi$ degrees in the E-plane and $\theta$ degrees in the H-plane?
• Are there any approximations to this?

Answer 1

If you're OK with an approximation, the solid angle can be calculated with much simpler math.

An isotropic antenna must radiate over a solid angle of 4π because it radiates in all directions.

An antenna with a gain of (for example) 20 dBi has a gain of 100x, which means in the direction that gain is quoted, the power flux density must be 100x what it would have been for an isotropic antenna.

If we assume the antenna achieves its gain by an ideal beam which has some equal intensity within the beam, and no radiation outside the beam, then calculating the solid angle is a simple matter of division:

$$ {4 \pi \over 100} = {0.04 \pi} $$

In practice a real antenna will have some sidelobes, meaning this method overestimates the beam solid angle. But also the quoted gain is at the peak, whereas the power flux density will on average be less than that, meaning this method underestimates the solid angle. A practical approach could call these errors a wash.